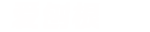1/1 x²
arctanx的导数是1/1 x²,设y=arctanx,则x=tany,因为arctanx′=1/tany′,且tany′=(siny/cosy)′=cosycosy-siny(-siny)/cos²y=1/cos²y,则arctanx′=cos²y=cos²y/sin²y cos²y=1/1 tan²y=1/1 x² 。
arctanx(即Arctangent)指反正切函数 。反函数与原函数关于y=x的对称点的导数互为倒数 。设原函数为y=f(x),则其反函数在y点的导数与f'(x)互为倒数(即原函数,前提要f'(x)存在且不为0) 。
反正切函数arctanx的导数
【arctanx的导数是什么 arctanx的图像】(arctanx)'=1/(1 x^2)
函数y=tanx,(x不等于kπ π/2,k∈Z)的反函数,记作x=arctany,叫做反正切函数 。其值域为(-π/2,π/2) 。反正切函数是反三角函数的一种 。
反正切函数arctanx的求导过程
设y=arctanx
则x=tany
因为arctanx′=1/tany′
且tany′=(siny/cosy)′=cosycosy-siny(-siny)/cos²y=1/cos²y
则arctanx′=cos²y=cos²y/sin²y cos²y=1/1 tan²y=1/1 x² 。
所以arctanx的导数是1/1 x² 。
其他常用公式
(arcsinx)'=1/√(1-x^2)
(arccosx)'=-1/√(1-x^2)(arctanx)'=1/(1 x^2)(arccotx)'=-1/(1 x^2)
推荐阅读
- 冰箱宽度一般是多少
- 小青龙怎么保持鲜活
- 国庆节etc进入人工窗口出来可以吗
- 天气晚来秋的上一句 天气晚来秋的前一句
- 水表倒转什么原因
- 烟火的说说 生活就是人间烟火的说说
- 晚上零下7度白菜会冻坏吗
- 梵西是哪个国家的
- 高铁有无座票吗